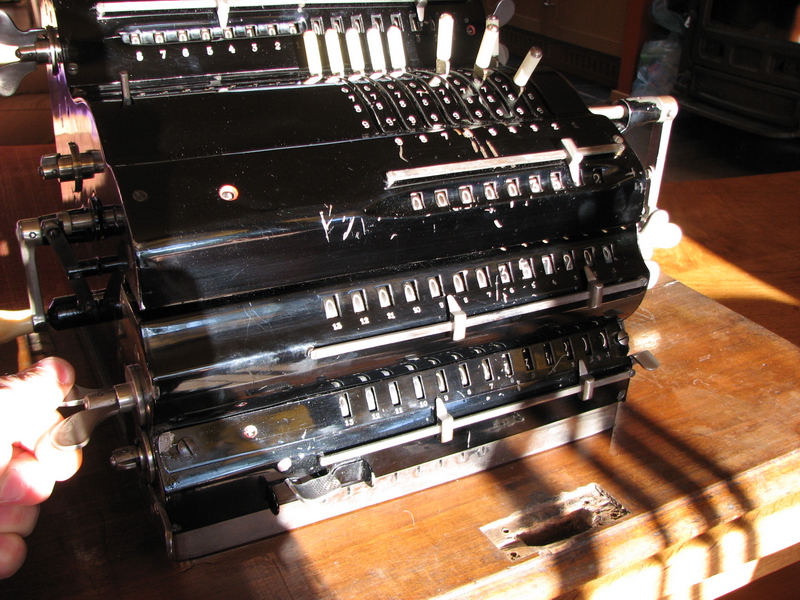
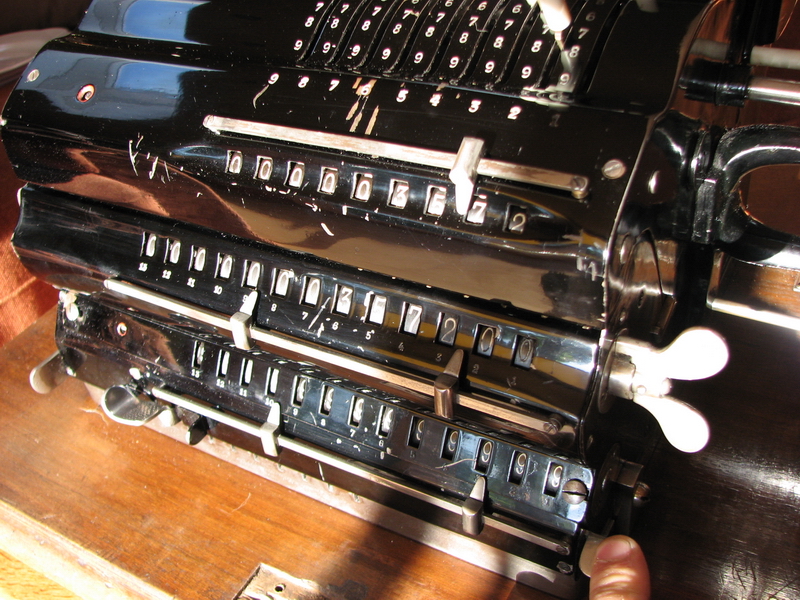
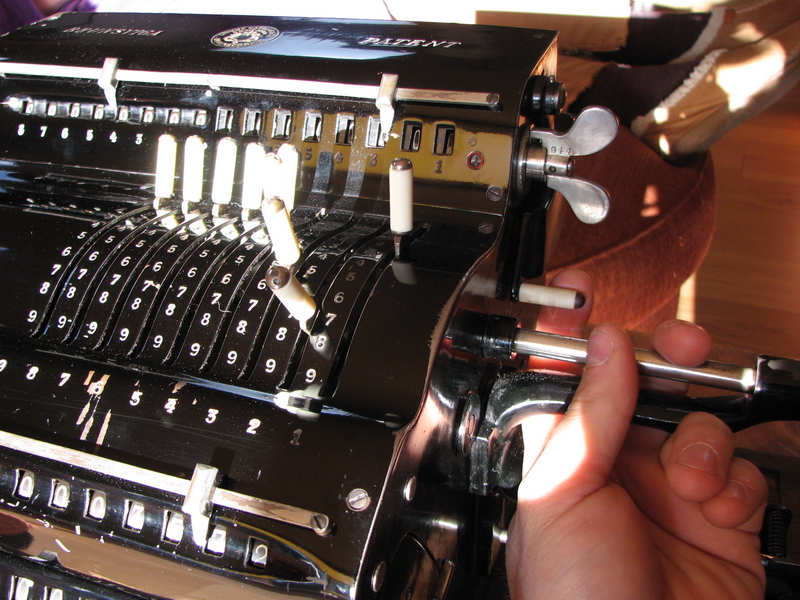
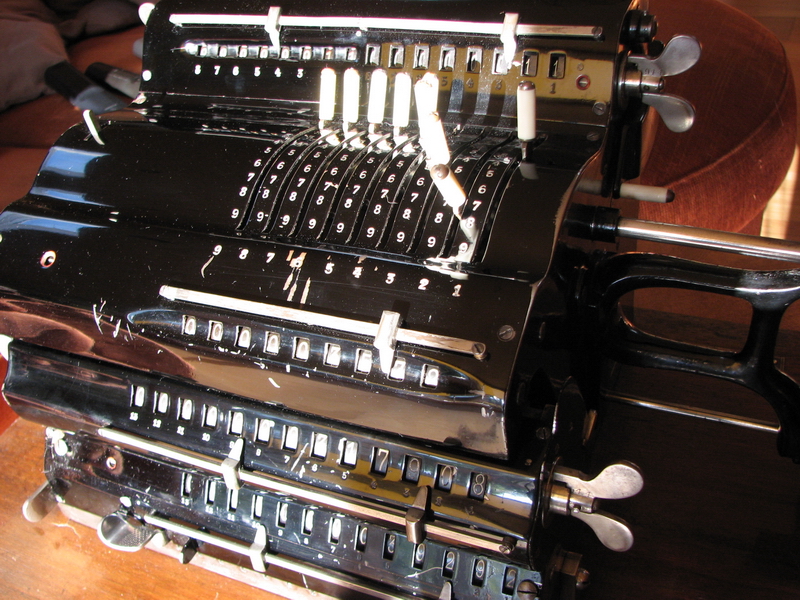
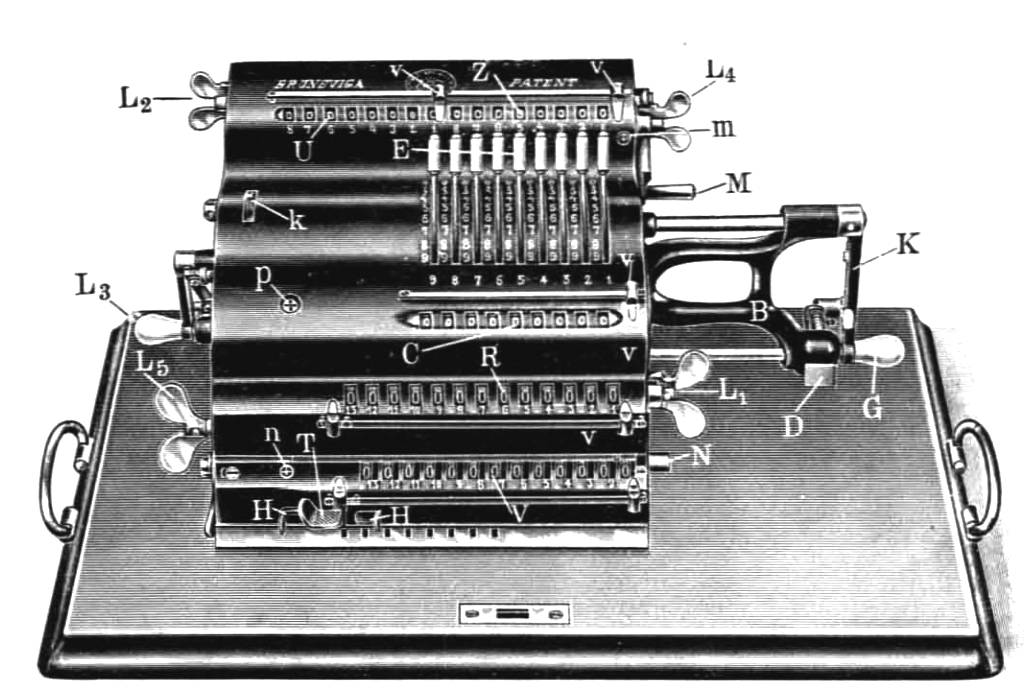
Brunsviga G
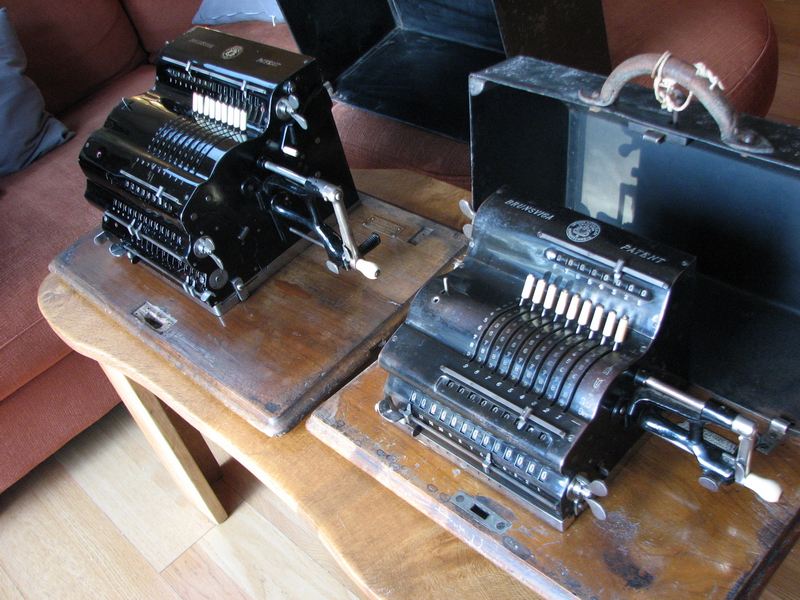
I have been so lucky to be the caretaker of two of these machines, and both came from the north of France. This version of the Brunsviga G is listed in Trautschold, and an example calculation of what it can do is reproduced from there at the end of this page.It was the absolute top model of the Brunsviga range in 1908 (together with the Arithmotyp). It had non-moving setting levers, like the H, J, and N models, and twin counter registers, one of which has full tens' carry, like the model H. But in addition, it also had an extra result register with provision for calculating with complements. Brunsviga advertised the machine as invaluable for product sums where the intermediate results had to be noted down, subtractive multiplication and percentage deduction. All manner of shortened multiplications were allowed by the counter register with tens' carry. The second result register can be switched to complements, as can the right revolution counter. There is a second revolution counter on the left, that has no tens' carry. This allows all sorts of interesting calculations in e.g. statistics, even though the Brunsviga promotional material does not really mention that, and perhaps only at the cost of not being able to use shortened multiplication. The only possible feature missing in the machine, which occurs only in the model N, is backtransfer from the result to the setting register.
The machine was built 97 times between 1907 and 1911. In that sense it can hardly be called a success. This example was made late in 1908 and sold by Neumann in Paris, probably to a very prestigious insurance firm or bank, because it was the most expensive Brunsviga that could be bought at the time, on par with the Trinks Arithmotyp, and priced at 1215 Mark. To give an idea of what this would be worth today, it was the equivalent of +/-3000 hours of unskilled labor. In 2015 Euro's, that would equal 65.000 EUR. In absolute worth, i.e. the price of goods in the index, 1215 Mark in 1908 equalled the equivalent today of 6000 EUR in goods, 14500 EUR in gold, or 7500 EUR in silver. Thanks to Rodney Edvinsson of Stockholm University, who made this historical currency converter.
Prices of other Brunsviga machines at the time (1910) were e.g.:
The Model G was thus more than twice as expensive as the Basic Model B, whereas there was also a 20% premium to be paid for a miniature machine 2/3rds the size!
What makes it even more mind boggling is that for this very limited series of 97 machines, the Brunsviga designers redesigned practically everything. Sure, it has a few parts in common with the rest of the non-moving setting lever range - but no more than a few, and then mainly gears, comma sliders, a clearing crank and lever handles and (some!) numeral wheels and such. The double carriage requires a pinwheel cylinder that is much larger than in an equivalent J or H machine - so this was entirely redesigned. All the castings are different. The revolution counter with tens' carry is a completely new development too (although common to the model H), as are all the bells and whistles in the double carriage, including the complements mechanism, which needs numeral wheels with two numbers per position, engraved in reverse order as they normally are, because the register rotates in the opposite of the normal sense. This is due to the presence of intermediate gears to bring it forward enough for the user to see. There are also many unique safeties built in, which will be encountered again in Brunsviga machines much later than 1908 - there is an interlock that prevents clearing the result registers when the carriage is not at its left stop. There is an interlock that prevents clearing the result registers when the pinwheel cylinder is not in its rest position, and the same is true for the revolution counters. The switch for setting the lower register to complements is also interlocked with the clearing and the carriage position both. All custom designed for this machine, and these parts won't fit anything else.
Known serial numbers for some of the 97 Brunsviga G machines that were built are 13933 and 13944. There was at least another one in the Brunsviga collection, under catalog nr. III Zrz 1923-9 or M548, and that was serial number 13154, although it is listed there as a Brunsviga MG (which is incorrect - the weight is 40kg, and the picture fits these machines to a T - the catalog entry also states that "only about 6 of these machines have been built", but if this were true and all the MGs in the Brunsviga catalog are piled in with the two I found, we would be up to 7 already, 3 of which are identical. There is a sidenote on the G/MG machines in the Brunsviga collection and elsewhere here.)
Haase's list gives 97 as the number built for the Brunsviga G, between 1907 and 1913.
The machines needed quite a bit of restoration. If you want to see how the transformation happened (very picture intensive!) you can do so following these two links for N° 13944 and 13933
We'll start with some pictures to show the machines - first n° 13944
Then n° 13933





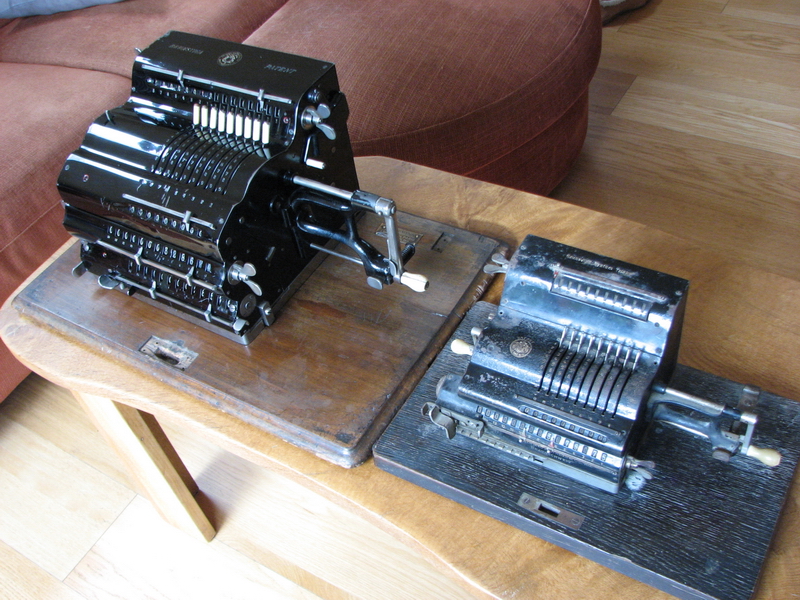
An interesting difference between the machines is the tabulator that the older machine has, but the newer one doesn't. N° 13154 in the Brunsviga catalog also has no tabulator. There is also a difference in the locking mechanism for the setting levers - on the newer machine it needs to be positively disconnected, on the other machine it disconnects itself as soon as the crank is pulled out. Why was this changed ? Who knows ... clearly these have been development machines for nearly all of the production run.
To give you an idea of the size of the machine, the whole thing weighs 35kg, and that is without the box, which adds another 3kg. If I have described the Brunsviga J as the Rolls Royce of calculators, well, then this thing is the V12 27 liter Meteor-engined blower Bentley. It is absolutely staggeringly massive. Here's a comparison with a "normal" Brunsviga J, which, at 14kg, looks decidedly tiny:
These are pictures with its even smaller sister, a (currently not yet restored) MJR.
Isn't it amazing that in the course of 16 years, from 1892 to 1908, Brunsviga managed to get from this:
to this :
That is an amazing amount of development!
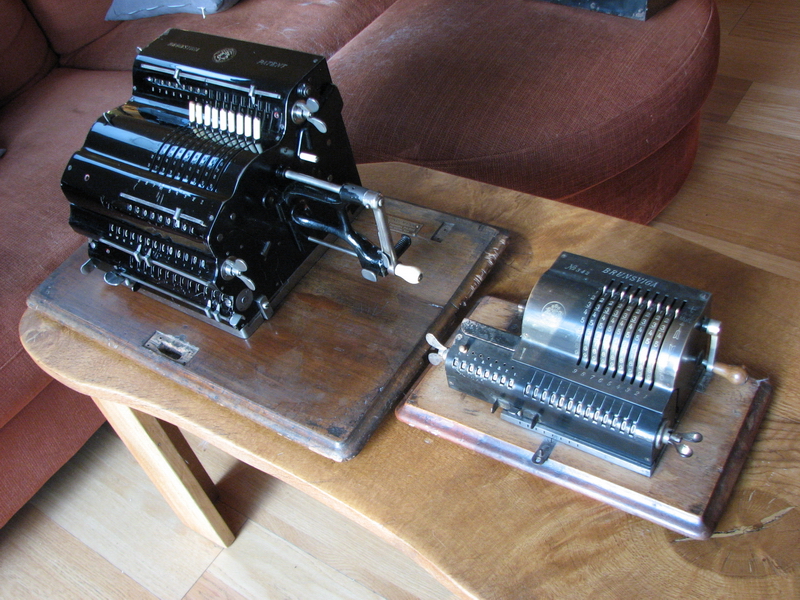
To complete the story, I made some pictures of the two sisters sitting side by side ... probably for the first time since 1908!



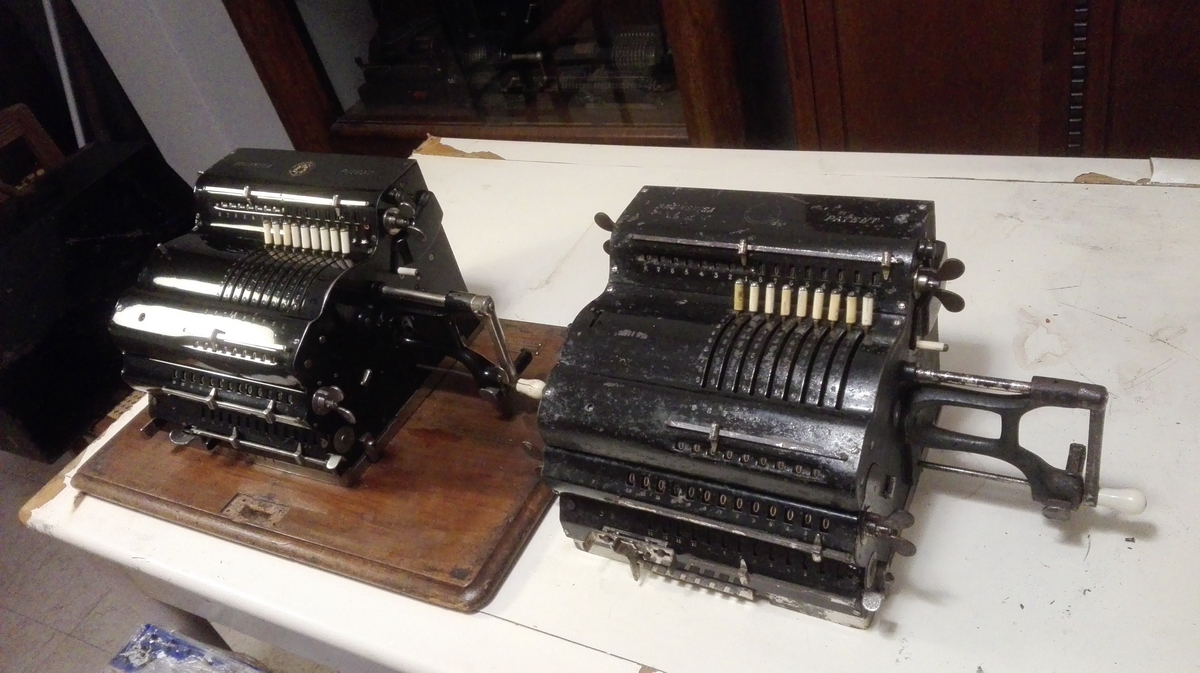

An interesting difference between the machines is the tabulator that the older machine has, but the newer one doesn't. There is also a difference in the locking mechanism for the setting levers - on the newer machine it needs to be positively disconnected, on the other machine it disconnects itself as soon as the crank is pulled out. Why was this changed ? Who knows ... clearly these have been development machines for nearly all of the production run.
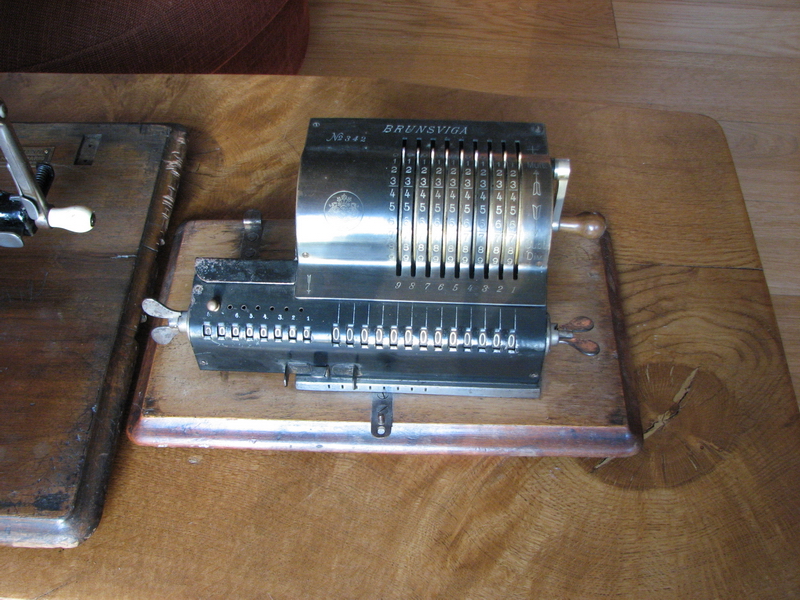
Now, on operation - the machine is not as versatile as one would expect, for the simple reason that the second register is not an additional memory with transfer from the first register, as in many other machines - it is a real result register, which is always in engagement with the pinwheel cylinder, and cannot be switched off. The registers can be cleared separately though, so this is quite useful for keeping tabs on single terms in a sum of products. The bottom register can be switched to negative, i.e. whatever you subtract will be counted in the positive sense in this register. Nice for having independent confirmation of the total amount subtracted from a value present in the top result register.
The twin counter registers are also not as useful as they potentially could be. The right one has tens' carry, the left one does not, so anything calculated by a shortened multiplication only makes sense in the right register anyway. Clearing the right register also clears the left register at the same time - but the left register can be cleared independently. Now, in order to demonstrate the capabilities of the machine, we will reproduce the calculations specifically outlined for the Modell G in Trautschold. As he writes (more or less verbatim):
Exercise XVI: Goods in a train station should be weighed and paid for before being loaded. We have:
How high is the total weight, and what is the total number of goods items ?
Machines with a counter without tens carry are unsuitable for this type of calculation - the total weight can be calculated, but the number of items cannot. (Note: actually, if, in addition to the weights, you set a 1 in the left part of the input register as an item counter, you can ...but Trautschold does not mention this, in order to sell more advanced machines to an unsuspecting clientèle ...). Here the advantages of the Models G and H are obvious, as they simultaneously allow two chain calculations.
What is the total weight and number of items of the various item categories ?
If all the numbers desired in this assignment are to be calculated in a single calculation, only the model G can be concerned. With this machine, one proceeds with the number results as in the previous assignment. The single results which appear in register "R", which in this case are of interest, are noted down, and the register cleared every time with L1. In the second result register "V", the sum of products appears, which should not be cleared, as the final sum gives the desired total weight. The total item count is given as well by the model H.
Subtractive multiplication calculations
Exercise XVII: 3.895.432 - 6.448 x 25
Set 3.895.432, 1 + turn, clear "U". For models G and H, "Z" can be cleared, or simply ignored.
Set 6.448, shift carriage left, 2 - turns.
shift carriage right, 5 - turns.
Result: 3.734.232
If "Z" was cleared after the initial setting, both "Z" and "U" give "25" in white numbers.
Exercise XVIII: 3.895.432 - 6.448 x 27
In regular machines, the assignment is calculated as above, but in the models G and H, shortened multiplication can be used:
Set 3.895.432, 1 + turn, clear "U" and "Z"
With the models G and H, before starting the subtractive multiplication - but only on the condition that shortened multiplication will be used- the minus lever "M" must be pulled forward, until it clicks into position. Due to the subtractive character of the rest of the calculation, it is only natural that the next turn of the crank must be in negative direction. Please beware of inadvertedly trying to complete a positive turn of the crank by force, as the mechanism that blocks the +-direction can be damaged. All the following turns of the crank can be executed in + or - direction according to the requirements of the calculation, and without an additional operation of the "M" handle. If doubt exists whether the counter "Z" was indeed set for subtractive multiplication at the start, one can check the +/- check window "m" for the - sign. If this should not appear, pull the "M" handle again until it makes its appearance. Set 6.448, shift carriage left, 3 - turns.
shift carriage right, 3 + turns.
Result: 3.721.336
In all models, the counter register "U" shows "33", counter register "Z" of the models G and H shows "27". If subtractive multiplication was executed without pulling the lever "M", counter register "Z" shows a useless result (sic!). (note: this can still be repaired by pulling the lever "M", and making a negative turn, followed by a corrective positive turn with the main crank - the counter will now show the correct number instead of a "useless result".)
All examples so far demonstrate the special role of the counter register "Z" in models G and H, as it shows the multiplier in any kind of shortened multiplication exactly as it features in the original problem.
Discount and other percentage calculations
Every calculation in which a certain percentage must be subtracted from a given number, is equivalent to a subtractive multiplication. The only difference is that the gross value that must be set into the machine is identical to the value to be subtracted afterwards. Other than that, the calculation follows the previous examples exactly. All that remains is to establish how to deal with the different decimal positions in gross value and percentage.
Exercise XIX: Of 3.572Mk, 3% must be subtracted.
Set 3.572, tab carriage to the right 2x, 1 + turn: 3.572,00. Mark decimal point with a marker.
Clear counter register. Shift carriage to the left 2x, 3 - turns: 3.464,84
Exercise XX: Now subtract 8%
The assignment is identical to the previous one, but can also be solved as subtractive multiplication on the models G and H with the use of the minus-lever "M". The resulting net value of 92% of 3.572 (3.286,24) is formed by subtracting 10% and then adding 2%.
Exercise XXI: Now subtract 8% - What is the net value, and what is the value of the discount in Mark ?
After solving the previous exercise, the result register in all models shows 3.286,24, the counter register the percentage discount as the number 12, and in the models G and H the percentage discount as 8, exactly as featured in the original assignment. The amount of the discount in Mark cannot be found, and must be calculated additionally. The model G has a special provision through which this number can be recovered without an additional calculation, in the following way:
Set 3.572. Shift carriage to the right 2x. 1 + turn. Clear both counter registers.
Shift carriage left 2x. Clear result register "V"
Now the switch "N" at the right side of the bottom register V is pressed, and the - sign appears in the check window "n".
The switch N must only be pressed for subtractive calculations, and only once. As soon as it is pressed, the next crank turn must be negative. All other turns of the crank can be executed in either direction, as the calculation requires. The further course of the calculation is to be executed as any other subtractive multiplication, and especially as the preceding example, with reference to the multiplier 8, as a shortened subtractive multiplication - thus:
Clear counter register "Z".
Pull lever "M to the front"
This is the counting register before pulling "M" - notice the indicator "m" at "+" and the shroud to the left -
And this is after, with the shroud to the right and the "-" indicator
1 - turn : R: 3.536,28, V: 35,72, U: 1, Z: 1
Two units were added to the counter on this one crank turn, through the special "M" mechanism.
shift carriage right, 1 - turn: R: 3.179,08, V: 392,92, U: 11, Z: 11
shift carriage left, 3 + turns: R:3.286,24, V: 285,76, U: 12, Z: 8
Result: net value Mk. 3.286,24, discount Mk. 285,76
At the same time, the setting check register shows the gross value of Mk. 3.572 (which is also the case for models J and H), and the percentual discount through the number 12 in "U" and 8 in "Z". The model G thus proves itself as an extremely efficient machine for percentual calculations.
(end quote by Trautschold)
Note: despite the advertised efficiency, the last exercise could theoretically be solved with two less turns of the crank, simply by skipping the first step, and performing the subtraction in the left position first, then tab back to the right and give two positive turns instead of three. However, doing it like that would run the user into trouble, as it is impossible to shift the carriage with the extra carry lever out. For technical reasons, not for logical ones, the message is - "press N, and whatever you do, immediately make a subtractive turn!" - lest the user would try otherwise and damage the machine.
Something Brunsviga didn't give a peep about at the time is the eminent usability of the machine for statistics. As already detailed in the page on the Triumphator CB, you could get, in one continuous operation, the sum and the sum of squares of a series of numbers, and with the second result register you could calculate the individual terms of the sum of squares as well. Very useful! To view an example of statistics with a machine with double result register, see my page on the Millionaire "Antonius". With the Brunsviga, however, you cannot do the same thing as with the Millionnaire, because the second result register cannot be disconnected from the machine, and the independent calculations of numbers minus their average can't be made without disturbing the sum of squares. If you do them by hand, or on a second machine (preferably a key driven comptometer!) it would work just fine.
To conclude with, if you are interested in the restoration process and the insides of these two machines, and found it lacking in the text above, you can follow these links for N° 13944 and 13933. Word of warnin gthough- they are very picture intensive.